Knowledge begins with God (Proverbs 1:7). But what is the mechanism by which we reason to have knowledge of other things? How does our knowledge that the “sun is bright” depend on revelation from God? By what chain of reasoning are we able to know anything about anything? How do we prove our most basic, foundational belief?
The Hierarchical Nature or Knowledge
We saw previously that knowledge is true, justified belief. To know something, we must believe it, it must be true, and we must have a good reason or several good reasons to believe it. These good reasons are the justification for the belief. But what constitutes a good reason? A good reason will be one that is itself true and justified. So unless the reason for a belief itself has a good reason, then the belief is ultimately unjustified. We must at some point ask, do we have a good reason to believe the reasons for our belief? And then, naturally, we will have to ask whether the reasons for our reasons themselves have reasons, and so on. Does this chain of reasoning go on forever?
An example may be helpful. Suppose I have the belief that the sun is bright. Is this knowledge? It is a true belief, but do I have a good reason to believe that the sun is bright? My reason might be that I have observed that the sun is bright with my eyes. That seems like a good reason. But it is only a good reason if I know that my eyes are reliable. So, do I have a good reason to believe that my vision is accurate? My reason for believing that my vision is accurate might be that I passed a vision test. Then of course, I will have to ask whether I have a good reason to believe that such a test is accurate. And so on.
Probability
Some of our knowledge is certain, but many of our beliefs are probabilistic in nature like a weather forecast. When we decide whether or not to bring a jacket or umbrella when traveling somewhere, we are making an educated guess about will happen based on what is most likely. When the forecast indicates a 95 percent chance of rain, we have a good reason to believe it will rain. But the belief is not certain. After all, there is a 5 percent chance that it will not rain. But what do these numbers really mean?
A 95% chance of rain today means that, in the past, when we examine all days that had measurable meteorological conditions comparable to today, it rained on 95 out of 100 of those days. Assuming that nature has some degree of underlying temporal uniformity, we can also conclude in the future on 95 out of 100 days that are like today, it will rain.[1] So, when there is a 95% chance of rain, the belief that “it will rain” is not guaranteed to be true; but it will be true 95 times out of 100. Going with the probability does not guarantee success. It simply means that, over time, you will be right more often than you will be wrong.
Many beliefs are based on multiple reasons. And the probability that the belief is true will depend on the probability that the reasons are true. Suppose my belief is “it was sunny yesterday.” What is the probability that my belief is actually true? My reason for believing this is that I remember going outside yesterday and I saw that it was sunny. So the belief “it was sunny yesterday” depends on the truth that (1) my vision is reliable AND (2) my memory of what I saw is reliable. Now suppose that I have a 90% chance that my vision is reliable, and a 90% chance that my memory is reliable. In that case, the probability that my belief “it was sunny yesterday” is 81%. It is less reliable than either of the reasons for it because they must both be true. And the probability that they are both true is the product of their individual probabilities (0.9 x 0.9 = 0.81). So, the greater the number of uncertain claims required for a belief, the lower the probability that it will be true.[2]
In everyday life, most of our assessments of probability are very subjective and imprecise. What is the exact probability that your brain has correctly processed the information transmitted from your eyes? We know it is less than certain because our visual perceptions can be fooled. Have you ever seen an optical illusion? Clearly, our sensory experiences are not 100% reliable in giving us an accurate picture of reality. They are basically reliable, but it’s hard to put an exact number on what the probability is.[3]
Nonetheless, in the Christian worldview we do have a good reason to believe that our senses are basically reliable – namely they were designed by God to inform our mind about the world. So we trust that they have some capacity to do that, though perhaps not perfectly because we are finite and because sin has corrupted our nature. Apart from the Christian worldview, people have a very difficult time justifying the basic reliability of sensory experience without begging the question. Namely, people often appeal to sensory experience as the basis for the reliability of sensory experience, which of course proves nothing.
Of course, some beliefs can be certain, in which case their probability is exactly 100%. In such cases, anything logically deduced from them will also be certain. “Laws of logic” also called “rules of inference” allow us to deduce truths from other truths. A proposition will be certain only if it is deduced from another proposition that is certain. Otherwise, it will have some lesser probability of being true depending on the probability of the truth claims upon which it is based. But all knowledge – whether certain or probabilistic – is based on a chain of propositions where the truth of one depends on the truth of others.
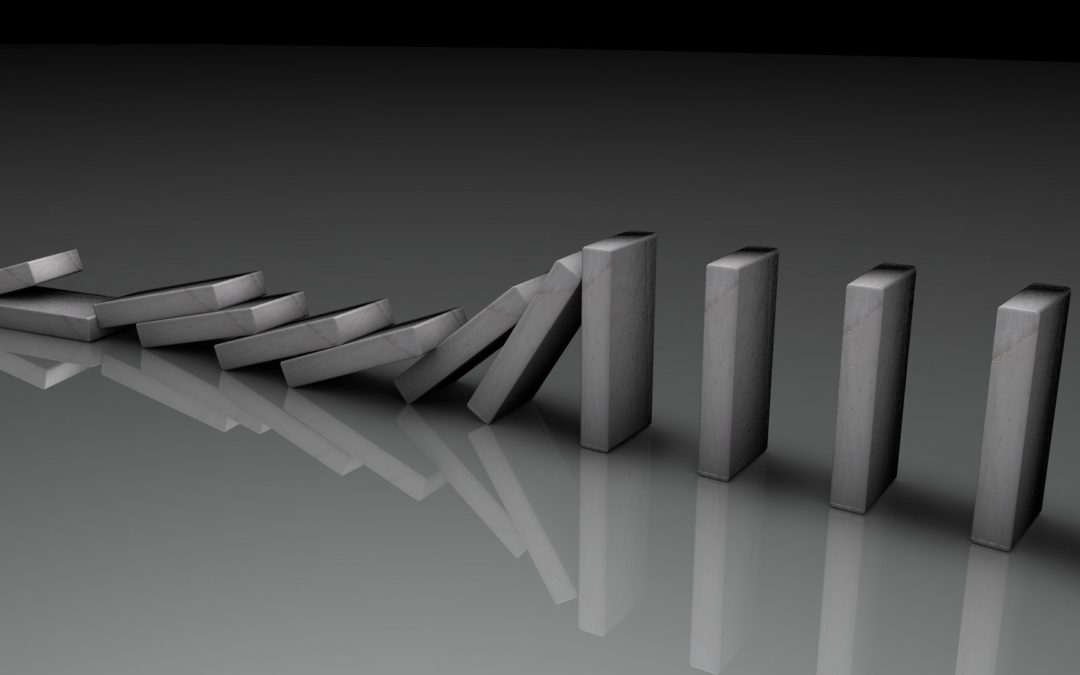
But we must eventually ask how far down the chain of reasoning goes. If I know truth “p” because it follows logically from truth “q” which follows logically from “r”, how do I know “r”? Perhaps “r” follows from “s”, but then how do I know “s”? Does this chain of reasoning ever end, or does it go on forever? If it ends in an ultimate foundation, then how do we know that this foundation is true?
The Münchhausen Trilemma
An old story involves Baron Münchhausen riding his horse when they became stuck in a swamp. The solution? The good Baron pulled up on his own hair, pulling both himself and his horse out of the muck. It’s impossible of course. You cannot pull on yourself to pull yourself up. Something external is required. And yet human knowledge seems to have basically the same problem. We know “p” because of “q” and “q” because of “r” and “r” because of “s”. But how do we know “s”? If the answer is “t” then we ask the same question of “t” and so on forever.
So, it seems that the chain of reasoning must go on forever. But it cannot go on forever because human beings are finite. We cannot know an infinite number of things, and therefore the chain of reasoning must end. That ending we might call an “ultimate standard” since there is no standard upon which it is based. But if it is not based on some other reason, then how is it justified? How do we know that the ultimate standard is true?
Some people might say “It’s obvious – self-evident.” One problem with this answer is that not everyone agrees on what this ultimate standard should be. So apparently, it’s not obvious or self-evident. Furthermore, many things that seem obvious are eventually discovered to be false. Others might say, “We cannot justify the ultimate standard. We simply must accept it.” But if the ultimate standard cannot be justified, then it might very well be false. In fact, if it is not justified, then there is literally no reason to accept it. And since all other beliefs are based on this ultimate standard, they would not be ultimately justified either. In that event, we could be wrong about everything we think we know. Nothing would be ultimately justified, and therefore we wouldn’t really know anything!
Others might say that there are two ultimate standards “A” and “B”. The justification for “A” is “B” and the justification for “B” is “A.” But this of course is circular reasoning. It commits the fallacy of begging the question since if both A and B are false then neither is a legitimate proof of the other. Alternatively, a person might say that the ultimate standard proves itself. But this also seems circular. How can “s” be the justification for “s” without begging the question?
So we are left with three equally unsatisfying options. (1) The chain of reasoning goes on forever and can therefore never be completed – making knowledge impossible. (2) The chain of reasoning terminates in an ultimate standard that cannot be justified, meaning all other beliefs (which are based on it) are ultimately unjustified – making knowledge impossible. (3) The chain of reasoning terminates in one or more ultimate standards that rely upon themselves for justification – a circular argument, which is ultimately arbitrary and unjustified – making knowledge impossible. This perplexing problem is known as the Münchhausen trilemma.
If the Münchhausen trilemma is correct, then we can demonstrate that knowledge is impossible. But, of course, this is instantly self-refuting. If we know that knowledge is impossible, then we do know something and hence knowledge is possible. Therefore, the Münchhausen trilemma must be flawed in some sense. But how? What is the solution? More to come.
[1] Technically, this applies only in the limit of an infinite number of sample days. That is, as the number of days with meteorological conditions similar to today approaches infinity, the fraction of them in which it rained approaches 0.95.
[2] Strangely, it is also possible to have a belief that is more probable than any one of the reasons it is based on – if any one of those reasons would independently establish the belief. Suppose the probability that any given person has reliable vision is 90%. Then the probability that my belief “it is sunny right now” can be no higher than 90% if I am the only one looking. But if two people independently “see the sun” right now, then the probability that it is sunny right now is actually 99%. However, no combination of probabilities (less than certainty) can ever establish certainty.
[3] Curiously, the analysis of probabilities presupposes the certainty of mathematical truths, such as the laws governing probability. Otherwise we could have no confidence in our probability assessment of anything else.